z-Test for Proportions, Two Samples (Jump to: Lecture | Video )

Let's perform a z-test for proportions, two samples: Researchers want to test the effectiveness of a new anti-anxiety medication. In clinical testing, 64 out of 200 people taking the medication report symptoms of anxiety. Of the people receiving a placebo, 92 out of 200 report symptoms of anxiety. Is the medication working any differently than the placebo? Test this claim using alpha = 0.05.

Steps for z-Test for Proportions, Two Samples
1. Define Null and Alternative Hypotheses 2. State Alpha 3. State Decision Rule 4. Calculate Test Statistic 5. State Results 6. State Conclusion

Let's begin.

1. Define Null and Alternative Hypotheses

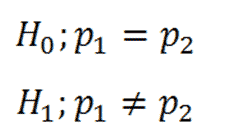
Figure 1.

2. State Alpha

Alpha = 0.05

3. State Decision Rule

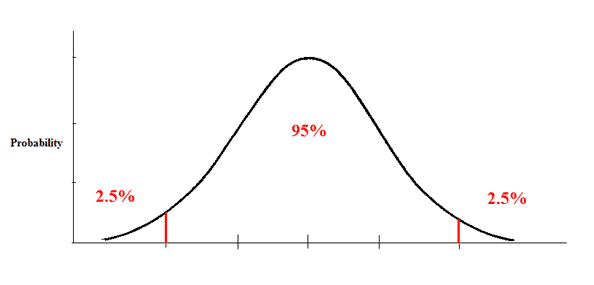
Using an alpha of 0.05 with a two-tailed test, we would expect our distribution to look something like this:

Figure 2.

Here we have 0.025 in each tail. Looking up 1 - 0.025 in our z-table, we find a critical value of 1.96. Thus, our decision rule for this two-tailed test is:

If Z is less than -1.96, or greater than 1.96, reject the null hypothesis.

4. Calculate Test Statistic

Figure 3.

5. State Results

z = 2.869

Result: Reject the null hypothesis.

6. State Conclusion

There was a significant difference in effectiveness between the medication group and the placebo group, z = -2.869, p < 0.05.

---