Wilcoxon Signed-Ranks Test (Jump to: Lecture | Video )

Wilcoxon Signed-Ranks Test
The Wilcoxon Signed-Ranks Test is a version of the dependent samples t-Test that can be performed on ordinal(ranked) data.

Ordinal data is displayed in the table below. Is there a difference between Before and After using alpha = 0.05?

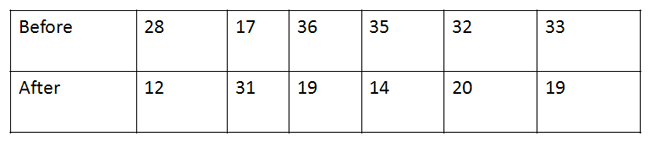
Figure 1.

Let's test to see if there is a difference with a hypothesis test.

Steps for Wilcoxon Signed-Ranks Test
1. Define Null and Alternative Hypotheses 2. State Alpha 3. State Decision Rule 4. Calculate Test Statistic 5. State Results 6. State Conclusion

1. Define Null and Alternative Hypotheses

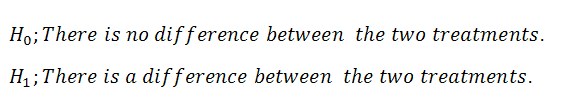
Figure 2.

2. State Alpha

alpha = 0.05

3. State Decision Rule

When you have a sample size that is greater than approximately 30, the Wilcoxon Signed-Ranks statistic follows the z distribution. Here, our sample is not greater than 30. However, I will still be using the z distribution for the sake of brevity. Keep this requirement in mind!

We look up our critical value in the z-Table and find a critical value of plus/minus 1.96.

If z is less than -1.96, or greater than 1.96, reject the null hypothesis.

4. Calculate Test Statistic

First, we must find the difference scores for our two groups:

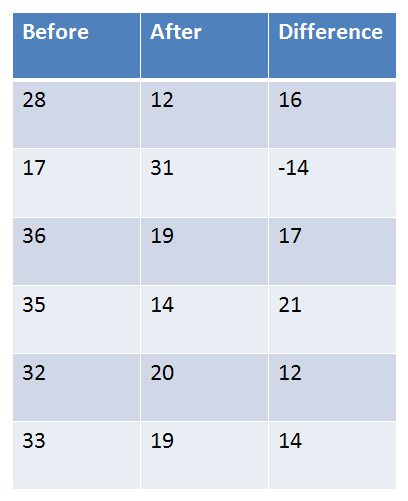
Figure 3.

Next, we rank the difference scores. Then, we add up the rankings of both the positive scores and the negative scores. We then take the smaller of those two values, which we call "T"

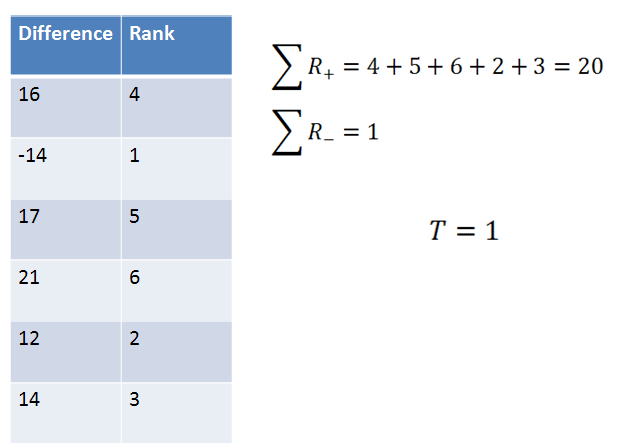
Figure 4.

The "T" score is then used to calculate the z statistic:

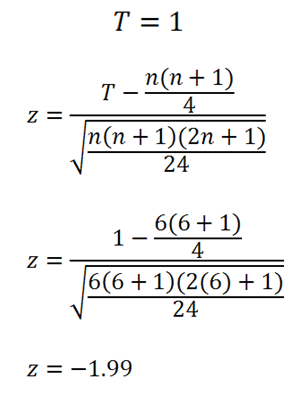
Figure 5.

5. State Results

If z is less than -1.96, or greater than 1.96, reject the null hypothesis.

z = -1.99

Reject the null hypothesis.

6. State Conclusion

There is a difference between the before and after groups, z = -1.99, p < .05.

---