Confidence Intervals for Independent Samples t-Test (Jump to: Lecture | Video )

We use the independent samples t-test to test if two sample means are different from one another. After the t-test, confidence intervals can be constructed to estimate how large that mean difference is.

Imagine we already have this data from a previous t-test:

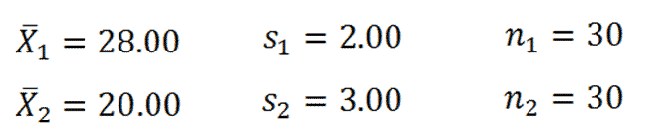
Figure 1.

Construct a 95% confidence interval for the difference of these two means.

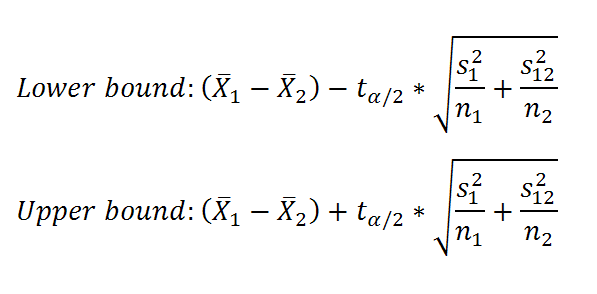
Figure 2.

Above are the equations for the lower and upper bounds of the confidence interval.

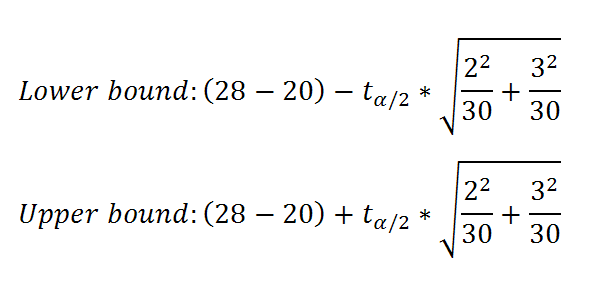
Figure 3.

We already know most of the variables in the equation, but what should we put for t? First, we need to calculate the degrees of freedom for both samples:

Figure 4.

Now, we'll use the smaller degree of freedom value to look up the t value. Go to the t-table and look up the critical value for a two-tailed test, alpha = 0.05, and 29 degrees of freedom. You should find a value of 2.0452. Now, we can finish calculating the lower and upper bounds:

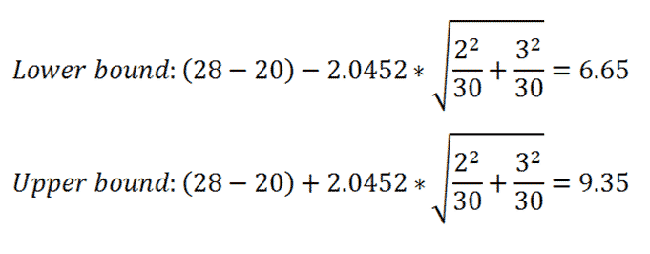
Figure 5.

We are 95% confident that the mean difference between sample 1 and sample 2 is between 6.65 and 9.35.